Sie sind hier: ICP » R. Hilfer » Publikationen

8 Fitting the excess wing of glass-forming glycerol

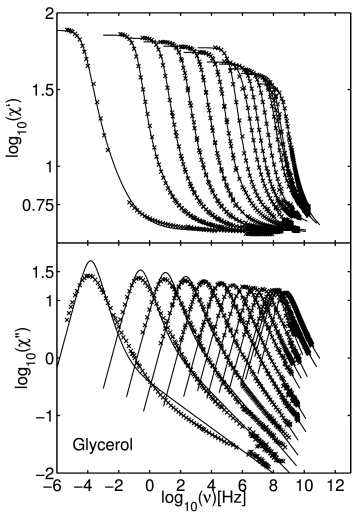
[405.1.2.1] In this section the composite fractional susceptibility (of type 0<μ<1) given in eq. (36) is used to fit broad band dielectric data of glycerol [43, 20]. [405.1.2.2] For more discussion of the experimental data see the contribution of P. Lunkenheimer and A. Loidl in this special issue.

[405.1.3.1] Figure 1 shows a fit to the experimental data of glycerol with the composite fractional susceptibility function given in eq. (36). [405.1.3.2] The upper figure displays the real part, the lower figure the imaginary part of the frequency dependent susceptibility χ. [405.1.3.3] The different curves belong to different temperatures ranging from T=323K down to T=184⁢K. [405.1.3.4] The normalized composite fractional susceptibility contains three fit parameters, while the traditionally used linear combination from eq. (27) contains six (resp. five when α2=1) fit parameters. [405.1.3.5] Because the experimental data are not normalized one additional parameter is needed in all cases to fit the data. [405.1.3.6] This extra paramter is the dielectric strength defined as

Δ⁢ε=χ⁢0-χ∞.(39)

[405.1.3.7] Figure 1 shows that not only the asymmetric α-peak but also the excess wing at high frequencies can be fitted quantitatively at all except the three lowest temperatures (T=204,195,184K) using the composite fractional susceptibility function (36) with only three essential fit parameters. [405.2.0.1] Note that for T=213K the fit extends over almost 9 decades in frequency.

Figure 1: Separate fits for χ′⁢ω (upper figure) and χ′′⁢ω (lower figure) using the composite fractional susceptibility from eq. (36) for temperatures T=323, 303, 295, 289, 273, 263, 253, 243, 234, 223, 213, 204, 195, 184 K (from right to left) as function of frequency ω=2⁢π⁢ν. The experimental data are taken from Ref. [43]. The corresponding fit parameters α,τ1,τ2 are shown in Figures 3 and 4. The dielectric strength Δ⁢ε is plotted in Figure 3 as function of temperature.

[405.2.1.1] If an iterated composite fractional time evolution [page 406, §0]    with four parameters is introduced an even better quantitative agreement can be obtained at all availaible temperatures. [406.1.0.1] In Figure 2 the composite fractional susceptibility

χ⌃⁢u=1+τ1⁢uα1+τ2⁢uα21+τ1⁢u+τ1⁢uα1+τ2⁢uα2(40)

with four parameters was used to fit the same data as in Figure 1. [406.1.0.2] This fit function has still two (resp. one) parameter less than the conventional fit function of eq. (27). [406.1.0.3] Note that in this case for T=184K the agreement extends over 13 decades in frequency including the full range of the excess wing.

Figure 2: Separate fits for χ′⁢ω (upper figure) and χ′′⁢ω (lower figure) using the composite fractional susceptibility from eq. (40) for temperatures T=323, 303, 295, 289, 273, 263, 253, 243, 234, 223, 213, 204, 195, 184 K (from right to left) as function of frequency ω=2⁢π⁢ν. The experimental data the same as in Figure 1.

[406.1.1.1] The values of the fit parameters were found to depend sensitively on the frequency range that was included in the fit. [406.1.1.2] For this reason real and imaginary part were fitted separately. [406.1.1.3] The variation of the fit parameters for real and imaginary part gives an impression of the quality of the fit. [406.1.1.4] One source for parameter variations might be that the experimental data are patched together from differentmeasurements. [406.2.0.1] The matching of different data sets leads to visible breakpoints in the experimental data sets.

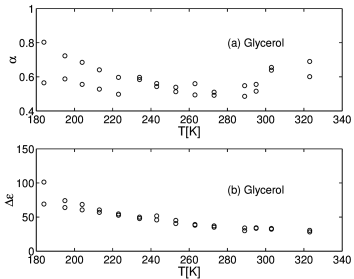
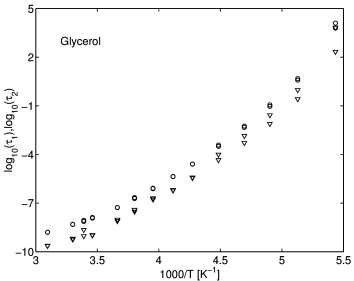
[406.2.1.1] In Figure 3 and 4 the fit parameters for real and imaginary parts corresponding to the fits shown in Figure 1 are plotted against temperature. [406.2.1.2] Figure 4 shows the relaxation times in an Arrhenius plot. [406.2.1.3] Clear deviations from Arrhenius behaviour are found. [406.2.1.4] Figure 3 shows the exponent α and dielectric strength Δ⁢ε from the normalized composite fractional susceptibility. [page 407, §0]    [407.1.0.1] Note that the dependence of α on temperature shows a qualitative different behaviour than for fits using Havriliak-Negami or Cole-Davidson functions. [407.1.0.2] In those cases the exponent decreases slowly with temperature from values around 0.8 to values around 0.5. [407.1.0.3] Here the values of α seem to remain flat for a temperature window between 200-300K where they fall into the range between α=0.5 and α=0.6. [407.1.0.4] The values seem to increase with lowering the temperature, but this could be an artefact because the low temperature fits are only qualitatively accurate. [407.1.0.5] On the other hand the increase at low T could also suggest a return to an effective non-fractional time evolution at low temperatures in the glassy phase. [407.1.0.6] For α→1 the excess wing in the composite fractional susceptibility function becomes increasingly flat.

Figure 3: (a) Stretching exponent α from eq. (36) for the fits shown in Figure 1. (b) Dielectric strength Δ⁢ε from eq. (39) for the fits based on eq. (36) shown in Figure 1. The two values at each temperature correspond to real and imaginary part χ′,χ⁢".
Figure 4: Relaxation times τ1 (circles) and τ2 (triangles) from eq. (36) for the fits shown in Figure 1. The two values at each temperature correspond to real and imaginary part χ′,χ′′.

[407.1.1.1] In summary the present paper has derived a novel three parameter susceptibility function from the theory of fractional time evolutions [3]. [407.1.1.2] The new function contains only a single stretching exponent. [407.1.1.3] It shows two widespread characteristics of relaxation spectra in glass forming materials: i) an asymmetry of the α-peak and ii) an excess wing at high frequencies. [407.1.1.4] The excess wingis not shown by the popular Cole-Cole, Cole-Davidson, Havriliak-Negami or Kohlrausch-Williams-Watts functions. [407.1.1.5] The new fit function with only three parameters yields agreement with broad band dielectric data over up to 9 decades in time. [407.1.1.6] A four parameter generalization gives good agreement over up to 13 decades in frequency. [407.1.1.7] Nevertheless the large uncertainty in the fit parameters indicates that smoother experimental data are needed to establish conclusively whether composite fractional time evolutions exist in experiment.