[68.1.2.1] This section returns to eq. (3.2)
and presents numerical solutions.
[68.1.2.2] This is intended as a case study exploring the relationship
between the statistics of local geometries
and bulk dielectric behavior.
[68.1.2.3] The main focus will be on dielectric enhancement.
[68.1.2.4] To solve eq. (3.2) for εω,
one must know the geometric input functions μϕ, λϕ
and local dielectric responses εCω;ϕ, εBω;ϕ.
[68.1.2.5] Unfortunately, no experimental data are available at present,[27]
and geometric modeling has to be used instead.
A Local dielectric response
[68.1.3.1] The hypothesis of local simplicity states
that the local geometries are simple
and that the effective local dielectric constants
are insensitive to geometrical details
other than local porosity.
[68.1.3.2] The simplest isotropic local
geometry is spherical.
[68.2.0.1] For conducting local geometries,
a water-coated spherical rock grain will serve
as the local model.
[68.2.0.2] For blocking geometries a rock-coated spherical
water pore is employed.
[68.2.0.3] In the notation of Sec. IV,
this means
| εCu;ϕ | =εW1-1-ϕ1-εR/εW-1-13ϕ, | | (6.1) |
| εBu;ϕ | =εR1-ϕ1-εW/εR-1-131-ϕ. | | (6.2) |
[68.2.0.4] In the low-frequency limit, one obtains for the conducting geometry
| σC′0;ϕ=σW′2ϕ3-ϕ=23σW′ϕ1+13ϕ+…, | | (6.3) |
thereby identifying C1 in eq. (4.8) as C1=23σW′.
[68.2.0.5] The real dielectric constant is found as
| εC′0;ϕ=εW′-1-ϕ1-13ϕ2εW′1-13ϕ-εR′. | | (6.4) |
[68.2.0.6] For the blocking geometry, the dc limit gives σB′0;ϕ=0,
in agreement with eq. (4.6), and
| εB′0;ϕ=εR′1+2ϕ1-ϕ, | | (6.5) |
for ϕ<1, identifying B1=1/εR′ in eq. (4.9),
and εB′0;ϕ=εW′, for ϕ=1.
[68.2.0.7] Note the presence of the thin-plate divergence in the ϕ→1 limit.
B Local porosity distribution
[68.2.1.1] It was mentioned repeatedly that no experimental data for μϕ
are available to the author at present.
[68.2.1.2] A qualitative guideline for porous media resulting
from spinodal decomposition might be the shape
of the order-parameter distribution calculated in Ref. [28]
which suggests in particular that μϕ can be bimodal.
[68.2.2.1] For the subsequent calculations,
a simple mixture of two β distributions has been used.
[68.2.2.2] The analytic expression reads
| μϕ=ωΓμ1+ν1Γμ1Γν11-ϕμ1-1ϕν1-1+1-ωΓμ2+ν2Γμ2Γν21-ϕμ2-1ϕν2-1, | | (6.6) |
where 0≤ω≤1, μ>0, ν>0 and Γx
denotes Euler’s Γ function.
[68.2.2.3] The bulk porosity is then given as
| ϕ¯=ων1μ1+ν1+1-ων2μ2+ν2. | | (6.7) |
[68.2.2.4] For μ,ν>1, the β densities are bell shaped,
and for μ,ν>1 they diverge at the limits.
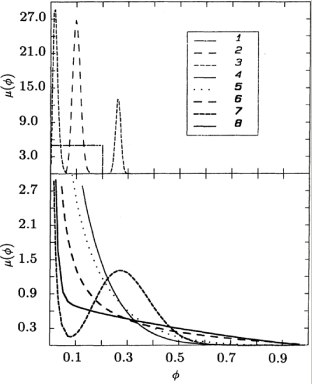
[68.2.3.1] Eight different local porosity distributions
are compared in the calculations.
[68.2.3.2] All of them are chosen such that they give
the same bulk porosity ϕ¯=0.1.
[68.2.3.3] The values of the parameters are listed in Table 1,
and the densities
[page 69, §0]
themselves are displayed graphically in fig. 1.
[69.1.0.1] Each distribution is identified by a number
and a line style as indicated in the inset of Fig. 1.
ϕ¯i (i=1,2) are the partial porosities
νi/νi+μi in eq. (6.7).
[69.1.0.2] The uniform distribution carries number 1
and is identified by a thin dot-dashed line.
[69.1.0.3] Number 2 represents the strongly peaked case
and is identified by a wide dashed line,
and so on.
Table 1: Paramenter values for the eight different forms of
μϕ
displayed in Fig.
1
and used in the model calculations.
The parameters
ω,
μ1,
ν1,
μ2 and
ν2
are those of Eq. (
6.6).
ϕ¯1,
ϕ¯2,
var1 and
var2
are the average and variance for
μ1,
μ2,
if
μ in Eq. (
6.6)
is written as
μ=ωμ1+1-ωμ2.
The rows labeled
ϕ¯, variance,
and skewness contain the mean, variance and skewness
for the eight forms of
μϕ.
|
|
|
|
|
|
|
|
|
| Curve No. |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
|
|
|
|
|
|
|
|
|
| ω |
1 |
1 |
2/3 |
1 |
1 |
1 |
2/3 |
2/3 |
| μ1 |
1.0 |
360.0 |
191.1 |
7.2 |
4.5 |
1.8 |
28.8 |
58.6 |
| ν1 |
1.000 |
40.000 |
3.900 |
0.800 |
0.500 |
0.200 |
0.087 |
0.176 |
| μ2 |
|
|
1423.0 |
|
|
|
13.9 |
2.24 |
| ν2 |
|
|
500.00 |
|
|
|
6.00 |
0.96 |
| ϕ¯1 |
0.1 |
0.1 |
0.02 |
0.1 |
0.1 |
0.1 |
0.003 |
0.003 |
| ϕ¯2 |
|
|
0.26 |
|
|
|
0.294 |
0.294 |
| var1 |
0.00333 |
0.00024 |
0.00010 |
0.00010 |
0.01500 |
0.03000 |
0.00010 |
0.00005 |
| var2 |
|
|
0.00010 |
|
|
|
0.01000 |
0.05000 |
| ϕ¯ |
0.1 |
0.1 |
0.1 |
0.1 |
0.1 |
0.1 |
0.1 |
0.1 |
| Variance |
0.00333 |
0.00024 |
0.01290 |
0.01000 |
0.01500 |
0.03000 |
0.02213 |
0.03541 |
| Skewness |
0 |
0.2657 |
0.6993 |
1.6004 |
1.8679 |
2.3124 |
1.1588 |
2.1176 |
[69.1.1.1] The choices presented in Fig. 1 are arbitrary.
[69.1.1.2] The reader should bear in mind, however,
that μϕ is easily measureable
and cannot be adjusted to fit experimentally
observed dielectric data when comparing
the theory with experiment.
[69.1.1.3] The choices for μϕ presented here are intended
as a case study illustrating different possibilities
that might occur in real or artificial experimental systems.
[69.2.0.1] The highly peaked μϕ (curve 2)
represents the limit of weak disorder.
[69.2.0.2] Remember that μϕ=δϕ-ϕ¯ for ordered systems.
[69.2.0.3] Curve 2 gives a reference to which other distributions can be compared.
[69.2.0.4] The distributions 4, 5 and 6 have been chosen divergent at ϕ=0
with exponents 0.8, 0.5 and 0.2 as examples for distributions
whose inverse first moment does not exist.
[69.2.0.5] Curves 3, 7 and 8 demonstrate the fact that μϕ
itself might be “of percolation type.”
[69.2.0.6] This occurs if the porous medium contains
two types of porosity or regions
of very different porosities.
[69.2.0.7] For curve 3 the ratio between the two porosities is roughly 100,
and the inverse first moment of μϕ exists.
[69.2.0.8] For curves 7 and 8, the ratio roughly 1000,
and the densities diverge at ϕ=0.
[69.2.0.9] In all cases the distributions were chosen critical
in the sense that the weight
for the higher-porosity component is 13.
C Local percolation probability
[69.2.1.1] The local percolation probabilities λϕ
can be measured simultaneously
with the local porosity distribution μϕ.
[69.2.1.2] However, such a measurement is more difficult
because it requires the approximate reconstruction
of the three-dimensional pore space from
parallel two-dimensional sections.
[69.2.1.3] For this reason geometric modeling of porous media
is most important for this quantity.
[69.2.2.1] Three simple models will be compared
in the calculations:
the uniformly connected model (UCM),
the central pore model (CPM),
and the grain consolidation model (GCM).
1 Uniformly connected models
[69.2.3.1] In these models λϕ equals a constant, i.e.,
[69.2.3.2] In the simplest case, the fully connected model, p=1.
[69.2.3.3] This means that all local geometries are assumed to be
[page 70, §0]
conducting.
[70.1.0.1] In addition, the case p=12 will also be investigated.
2 Central pore model
[70.1.1.1] Consider a cubic cell of volume 1 filled with rock.
[70.1.1.2] Inside the cubic cell a centered cubic pore
of side length a (0≤a≤1) is cut out.
[70.1.1.3] Now a random process is used to drill cylindrical pores
with square cross section from the faces of the cube toward the central pore.
[70.1.1.4] Sometimes these pores will connect to the central pore,
and sometimes not.
[70.1.1.5] The random process starts with the choice
of an arbitrary face of the cube.
[70.1.1.6] Now choose a random number r between 0 and 12.
[70.1.1.7] If r>121-a, a pore with square cross section
of side length b (0≤b≤a) is drilled
from the center of the face all the way to the central pore.
[70.1.1.8] The central pore had volume a3,
and the connection pore has the volume 12b21-a.
[70.1.1.9] If the random number fulfills f<121-a,
then the face is not pierced,
but instead the same volume 12b21-a
is removed from the wall in such a way
that the resulting pore space remains disconnected
from the pore space connected to the central pore.
[70.1.1.10] This process is repeated for all six faces of the cube.
[70.1.1.11] The cubic symmetry is not essential,
and a model with different symmetry can be defined similarly.
[70.1.2.1] The result of the process described above
is a cubic cell whose porosity can be expressed
in terms of the side length a of the central pore
and the ratio R=b/a as
| ϕ=a3+3b21-a=a31-3R2+3R2a2. | | (6.9) |
[70.1.2.2] According to the definitions in Section II,
the cell is called percolating
if there exist at least one path
within the pore space connecting a face to a face
different than itself.
[70.1.2.3] To obtain λϕ the probability
that either no or exactly one face
is pierced has to be calculated.
[70.1.2.4] This probability euqals 1-λϕ.
[70.1.2.5] Clearly,
| 1-λ=1-a6+a1-a5=1-a5. | | (6.10) |
[70.1.2.6] Thus, in the central pore model,
where aϕ,R is that root of eq. (6.9),
which fulfills 0≤a≤1 for all 0≤ϕ≤1 and 0≤R≤1.
[70.1.2.7] For R=1, i.e., b=a, it follows that ϕ∝a2
and thus a∝ϕ1/2,
resulting in λϕ∝ϕ1/2 for small ϕ.
[70.1.2.8] On the other hand, for R→0 one finds a∝ϕ1/3
for ϕ→0 and thus λϕ∝ϕ1/3 for ϕ→0.
[70.1.2.9] Thus the general conclusion for the central pore model is that
where γ can range between 12 and 13.
3 Grain consolidation model
[70.1.3.1] The grain consolidation model was proposed
as a simple geometrical model for diagenesis.[11][70.1.3.2] Its main observation is the existence
and smallness of the percolation threshold
in regular and random bead packings
when the bead radii are increased.
[70.1.3.3] In fact, the model has recently
been modified such that the critical porosity at which
conduction ceases can be arbitrarily small.[12][70.2.0.1] For regular bead packings, this implies
| λϕ=0forϕ<ϕc1forϕ>ϕc. | | (6.13) |
[70.2.0.2] For random packings λϕ will be smoothed out around ϕc.
[70.2.0.3] For simplicity, in this paper eq. (6.13)
will be used with
uphic=0.05.
[70.2.1.1] The most important aspect of λϕ
is that it determines the control parameter p.
[70.2.1.2] According to eq. (6.12),
its behavior near ϕ=0 can influence
the exponent α in (5.10c).
[70.2.1.3] Note that for the grain consolidation model
the form of λϕ always implies
that condition (5.10a) is fulfilled,
and universal behavior is expected.
[70.2.1.4] The half-connected model in the uniformly
connected model class is included
to demonstrate the influence of the thin-plate effect.
[70.2.1.5] The shape of λϕ in all other cases
gives extremely small probability
to blocking geometries with high porosities.
[70.2.1.6] This is expected to be generally true for interparticle porosity.
[70.2.1.7] This is expected to be generally true
for interparticle porosity.
[70.2.1.8] However, the secondary pore space in real rocks
may contain a significant fraction
of high-porosity blocking geometries.[32]
E Discussion
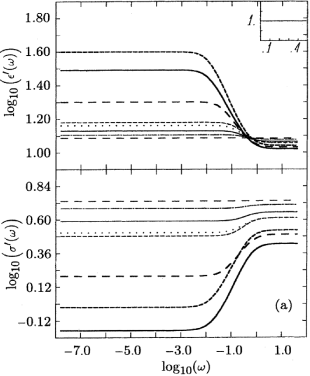
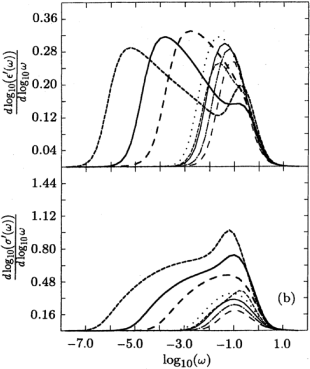
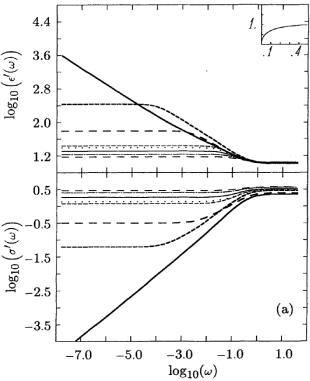
[70.2.3.1] It is obvious from Figs. 2-5 [especially part (b)]
that the low-frequency dielectric response
depends sensitively on the details of μϕ and λϕ.
[70.2.3.2] A general discussion is difficult,
because the response is always a mixture
between three basic mechanisms each of which can give
significant dielectric dispersion.
[page 71, §0]
[71.1.0.1] The first mechanism is the dispersion
resulting from the disorder in μϕ itself.
[71.1.0.2] The second mechanism is the despersion resulting from p,
i.e., from percolation geometry.
[71.1.0.3] The third mechanism is the dispersion
resulting from the behavior of λϕ
in the ϕ→1 limit, i.e., the thin-plate effect.
[71.1.1.1] The absolute dispersion for all figures is collected
in Table 2.
[71.1.1.2] Δε is defined as Δε=ε′0-ε′∞,
while Δσ=σ′∞-σ′0.
[71.2.1.1] Before discussing the three mechanisms,
it is important to note that the bulk porosity ϕ¯
does not influence the shape of the response curves
if it is changed without changing the shape of μϕ.
[71.2.1.2] Instead, it determines an overall frequency shift
for the frequency region over which the dispersion occurs.
[71.2.1.3] As ϕ¯ is lowered,
this region is shifted toward lower frequencies.
[71.2.1.4] This observation together with the fact
that all μϕ give the same bulk
porosity of
0.1
shows
that the bulk porosity by itself cannot be used
to characterize the dielectric response.
[71.2.1.5] In particular, there is no theoretical basis
for Archie’s law [eq. (5.27)]
if interpreted as a relation between
dc conductivity and bulk porosity
(see Section V.D for a discussion).
[page 73, §0]
[73.1.1.1] A second observation is that in all figures
the high-frequency real dielectric constant ε′∞
is not very sensitive to the details of μϕ.
[73.1.1.2] This is a consequence of the fact
that for low ϕ¯
the local dielectric constants εB′
and εC′ must both approach εR′.
[73.1.2.1] The first mechanism, dispersion from the form of μϕ,
can be studied in pure form when λϕ=1,
and the corresponding results are shown in Figure 2.
[73.1.2.2] In this case there are no blocking local geometries;
i.e., p=1 according to eq. (5.7).
[73.1.2.3] If μϕ is highly peaked as in curve 2,
then the system is only weakly disordered,
and there is almost no visible disperion
with the amount of disorder
contained in μϕ.
[73.1.2.4] In fact, distributions with power-law
divergences at ϕ→0 or with percolation structure
generate the strongest dispersion,
as can be seen from curves 3 and 5-8 in figure 2.
[73.1.2.5] Table 2 shows that the dispersion
varies almost three orders of magnitude
between the different distributions.
[73.1.2.6] Note that relatively similar
local porosity distributions
such as curves 6 and 8
can have very different dielectric response.
[73.1.2.7] On the other hand, very different shapes
for μϕ can give similar εω,
as demonstrated by curves 3 and 5.
[73.1.2.8] This shows that the dielectric response by itself
does not contain a full geometric characterization
of the pore space,
and it needs always to be complemented
with additional physical or geometrical information.
[73.1.2.9] This is not too surprising.
[73.1.2.10] Indeed, it is more surprising
that when the dielectric response becomes large
it is also very sensitive to geometric details.
[73.1.2.11] This is the case for dielectric enhancement
near the percolation threshold
or as a result of the thin-plate effect.
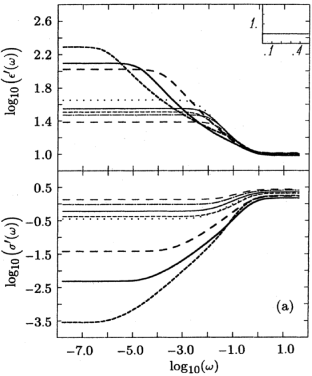
[73.1.3.1] Consider the thin-plate mechanism.
[73.1.3.2] It requires the presence of blocking geometries
of high porosity.
[73.1.3.3] Mathematically, this means λϕ≠1 for large ϕ.
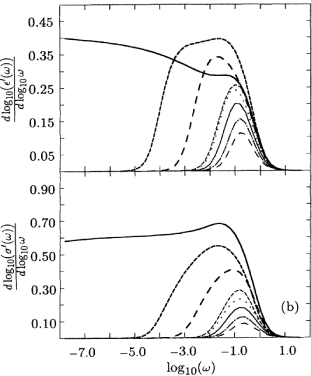
[73.1.3.4] As a simple illustration, Figure 3 displays the results
for the uniformly connected model
when λϕ=12.
[73.1.3.5] Now p=12, which is far away from pc.
[73.1.3.6] Nevertheless, the dielectric dispersion
is much stronger than would be obtained
for solutions to the central pore model
or grain consolidation model with the same p.
[73.1.3.7] Compare, e.g., curve 5 in figure 3
with curve 5 in figure 5.
[73.1.3.8] Moreover, the dielectric dispersion
becomes sensitive to the details μϕ.
[73.1.3.9] It is now possible to distinguish in figure 3(b)
the distributions 3, 7 and 8,
which have ω≠1
from the rest for which ω=1.
[73.1.3.10] In particular, curves 3 and 5,
which had very similar response in figure 2,
appear now very different.
[73.1.3.11] The dispersion is the stronger
the more weight μϕ has at high ϕ.
[73.1.3.12] This can be seen from curve 3,
which shows less dispersion than curves 4 and 5,
while the opposite was true for figure 2.
[73.2.0.1] Similarly, ε′ for curve 7
is depressed below curves 6 and 8 at intermediate frequencies.
[73.2.0.2] At very low frequencies,
the percolative character of distribution 7
is responsible for stronger overall dispersion
than in curve 6.
[73.2.0.3] The degree of asymmetry of μϕ
is reflected in the asymmetry of the response,
as best seen in the derivatives plotted in figure 3(b).
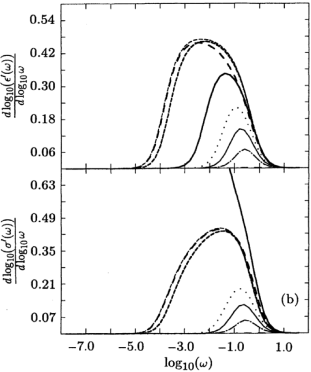
[73.2.1.1] The percolation mechanism is responsible
for strong dielectric disperion
in figures 4 and 5.
[73.2.1.2] There is essentially no dispersion
from thin-plate mechanism in these cases
because in both cases λϕ≈1 for
ϕ>0.5,
and thus there are no local geometrics with
a high dielectric constant.
[73.2.1.3] Figure 4 represents the central
pore model with
R=0.922,
and results for the grain consolidation model
with
ϕc=0.05
are given in figure 5.
[73.2.1.4] Contrary to the situation in figures 2 and 3,
p is now different for each distribution.
[73.2.1.5] The results of performing the integral
in eq. (5.7) are listed in table 3.
[73.2.1.6] Naturally, the dielectric dispersion
increases strongly with p→pc
and this effect dominates the dispersion from μ itself.
[73.2.1.7] In particular, for p≈pc
power-law behavior for εω
as a function of frequency is obtained
in agreement with the scaling theory
presented in Section V.
[73.2.1.8] As an example, scaling theory
predicts the exponent
0.597
for the conductivity of distribution 8 in figure 4 and
0.403
for the real dielectric constants.
[73.2.1.9] These predictions are obtained
from eqs. (5.23) and (5.24)
using s=1 and eqs. (5.13b)
and (6.12) with
γ=0.5,
and the exponent ν1 from Table 1.
[73.2.1.10] Figure 4(b) shows that these values
are indeed approached at low frequencies.
[73.2.1.11] Similarly, scaling theory predicts the exponent 0.5
for ε′ and σ′ corresponding
to distributions 3, 6 and 7 in figure 5.
[73.2.1.12] Again, these values are approached
as seen fom figure 5(b),
although the power-law behavior
occurs over a limited frequency range
because p is not sufficiently
close to the critical region.
[73.2.1.13] Note that curve 8 in figure 5
has dropped below the percolation threshold,
and thus the conductivity increases as ω2 for small ω.
Table 2: Numerical values for
Δε=ε′0-ε′∞
and
Δσ=σ′∞-σ′0
contained in the model calculations of Figures
2-
5.
|
|
|
|
|
|
|
|
|
| Curve No. 1 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
|
|
|
|
|
|
|
|
|
| Δε (Fig. 2) |
0.798 |
0.053 |
3.716 |
1.863 |
3.147 |
9.261 |
28.793 |
20.484 |
| Δσ (Fig. 2) |
0.298 |
0.035 |
1.067 |
0.619 |
0.889 |
1.545 |
2.400 |
2.029 |
| Δε (Fig. 3) |
19.197 |
13.819 |
21.956 |
25.162 |
34.950 |
96.046 |
186.010 |
115.270 |
| Δσ (Fig. 3) |
1.585 |
1.370 |
1.664 |
1.674 |
1.741 |
1.648 |
1.751 |
1.489 |
| Δε (Fig. 4) |
5.996 |
3.835 |
16.616 |
9.639 |
14.204 |
52.196 |
259.700 |
3932.522 |
| Δσ (Fig. 4) |
1.105 |
0.801 |
1.974 |
1.455 |
1.708 |
2.176 |
2.877 |
2.283 |
| Δε (Fig. 5) |
2.119 |
0.053 |
371.525 |
5.498 |
11.430 |
291.481 |
277.396 |
37.691 |
| Δσ (Fig. 5) |
0.595 |
0.035 |
3.025 |
1.137 |
1.604 |
2.565 |
3.043 |
2.436 |
[73.2.2.1] The complexity and variability of εω
obtained from the simple mean-field solutions
of this section correspond to the complexity
and variability of possible pore-space geometries.
[73.2.2.2] More approximate analytical investigations
of the solutions to eq. (3.2)
are necessary to identify simple parameters
characterizing μ and λ
which allow a better classification
of the solutions and thereby the possible geometries.
Table 3: Calculated values of
p
corresponding to the eight different forms of
μϕ
for the central pore model (CPM, displayed in Figure
4)
and for the grain consolidation model
(GCM, displayed in Figure 5).
Note that
p=1 for all cases in Figure
1
and
p=12 for all cases displayed in Figure
2.
|
|
|
|
|
|
|
|
|
| Curve No. |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
|
|
|
|
|
|
|
|
|
| CPM |
0.6542 |
0.6940 |
0.5420 |
0.5858 |
0.5341 |
0.4059 |
0.3643 |
0.3334 |
| GCM |
0.7500 |
1.0000 |
0.3399 |
0.6048 |
0.4962 |
0.3415 |
0.3376 |
0.2841 |