I.A The Problem
Almost all studies of transport and relaxation in porous media are motivated by one central question. How are the effective macroscopic transport parameters influenced by the microscopic geometric structure of the medium ?
My presentation will divide this central question into three subproblems. The first subproblem is to give a quantitative geometric characterization of the complex porous microstructure. It will be discussed in chapter II and III. The second subproblem, treated in chapter IV and V, is to calculate effective macroscopic transport properties from the geometric characterization and the equations of motion for the phenomenon of interest. The third subproblem, known as the problem of “upscaling”, runs as a common thread through all chapters, and consists in defining and controlling the macroscopic limit. The macroscopic limit is a limit in which the ratio of a typical macroscopic length scale (e.g. the sample size) to a typical microscopic length scale (e.g. grain or pore size) diverges.
Distinguishing between the first problem and the second problem is conceptually convenient and important. Geometric properties of porous media are determined exclusively by the complex system of internal boundaries which defines the microstructure. Geometric properties can be calculated from a complete specification of the microstructure alone. Physical transport and relaxation properties on the other hand require in addition exact or approximate equations of motion describing the physical phenomenon of interest. Often this involves the relaxation of small perturbations or the steady state transport of physical quantities such as mass, energy, charge or momentum. The distinction between geometric and physical properties of porous media has become blurred in the literature [1, 2], and physical transport properties such as fluid flow permeability or formation factors are sometimes referred to as geometric characteristics. This can be understood because practically employed experimental methods for observing the pore space geometry often involve observations of relaxation and transport phenomena from which the geometry is inferred by inversion techniques.
Geometric properties of porous media are observed in practice either directly using light microscopy, electron microscopy or scanning tunneling microscopes (STM), or indirectly from interpreting experimental measurements of transport and relaxation processes such as fluid flow, electrical conduction, mercury intrusion or small angle x-ray and neutron scattering (SAXS/SANS). An overview over commonly used methods is given in Figure 1.
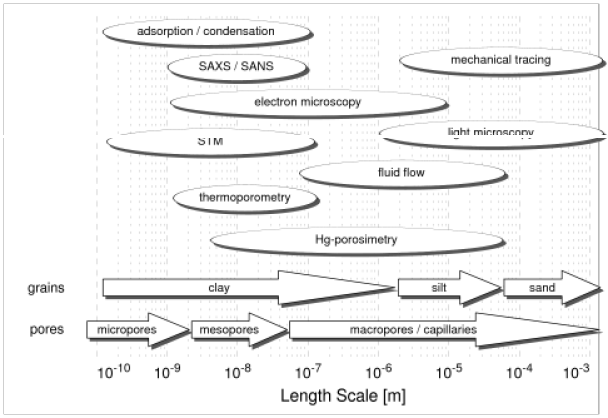
The different methods are represented on a length scale grid to indicate their ranges of applicability. The arrows in the lower part of figure 1 represent the IUPAC recommendation for classifying porous media into microporous, mesoporous or macroporous media, and the DIN classification of porous media into clay, silt and sand according to the grain size rather than pore size. Granular media with grains larger than 1mm are called gravels or boulders.
Loosely speaking a porous medium may be characterized as a medium containing a complex system of internal surfaces and phase boundaries. These internal interfaces define pores with a finite pore volume [3] and frequently a large surface area. Such a rough characterization applies also to other heterogeneous media and composites. Therefore some authors restrict the definition of porous media by requiring permeability [2], connectedness [4, 5] or randomness [5] as defining properties for porous media. Others [6, 7, 8], however, generalize the definition by including liquids into the purview. The precise definition of porous media adopted for the present review will be given below in section II.B.
