Sie sind hier: ICP » R. Hilfer » Publikationen

Appendix A Definition of H-Functions

The H-function is defined as [43]

H^{{m,n}}_{{P,Q}}\left(z\middle|\begin{array}[]{ccc}(\alpha _{1},A_{1})\cdots(\alpha _{P},A_{P})\\ (\beta _{1},B_{1})\cdots(\beta _{Q},B_{Q})\end{array}\right)=\frac{1}{2\pi i}\int _{{\mathfrak{C}}}\frac{\prod^{m}_{{j=1}}\Gamma(\beta _{j}-B_{j}s)\prod^{n}_{{j=1}}\Gamma(1-\alpha _{j}+A_{j}s)}{\prod^{Q}_{{j=m+1}}\Gamma(1-\beta _{j}+B_{j}s)\prod^{P}_{{j=n+1}}\Gamma(\alpha _{j}-A_{j}s)}z^{{-s}}ds, (A.1)

[p. 2474l, §2]
where \mathfrak{C} is a contour from c-i\infty to c+i\infty separating the poles of \Gamma(\beta _{j}-B_{j}s), j=1,\dots,m from those of \Gamma(1-\alpha _{j}+A_{j}s), j=1,\dots,n. Empty products are interpreted as unity. The integers m,n,P,Q satisfy 0\leq m\leq Q and 0\leq n\leq P. The coefficients A_{j} and B_{j} are positive real numbers and the complex parameters \alpha _{j}, \beta _{j} are such

[p. 2474r, §2]
that no poles in the integrand coincide. If

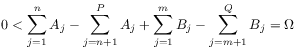0<\sum^{n}_{{j=1}}A_{j}-\sum^{P}_{{j=n+1}}A_{j}+\sum^{m}_{{j=1}}B_{j}-\sum^{Q}_{{j=m+1}}B_{j}=\Omega (A.2)

then integral converges absolutely and defines the H-function

[p. 2475l, §0]
in the sector \lvert z\rvert<\tfrac{1}{2}\Omega\pi. The H-function is also well defined when either

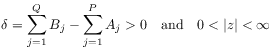\delta=\sum^{Q}_{{j=1}}B_{j}-\sum^{P}_{{j=1}}A_{j}>0\quad\text{and}\quad 0<\lvert z\rvert<\infty (A.3)

or

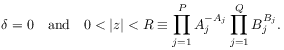\delta=0\quad\text{and}\quad 0<\lvert z\rvert<R\equiv\prod^{P}_{{j=1}}A_{j}^{{-A_{j}}}\prod^{Q}_{{j=1}}B_{j}^{{B_{j}}}. (A.4)

[p. 2475r, §0]
The H-function is a generalization of Meijer’s G function and contains many of the known special functions. In particular Mittag-Leffler and generalized Mittag-Leffler functions are special cases of the H-function.