Worldwide largest
threedimensional strongly correlated microstructure
with up to 35 184 372 088 832 discrete volume elements
and resolutions covering three decades from 117 µm down to 458 nm
Digital threedimensional images of unprecedented
 size and accuracy are available to the scientific
community from this website.
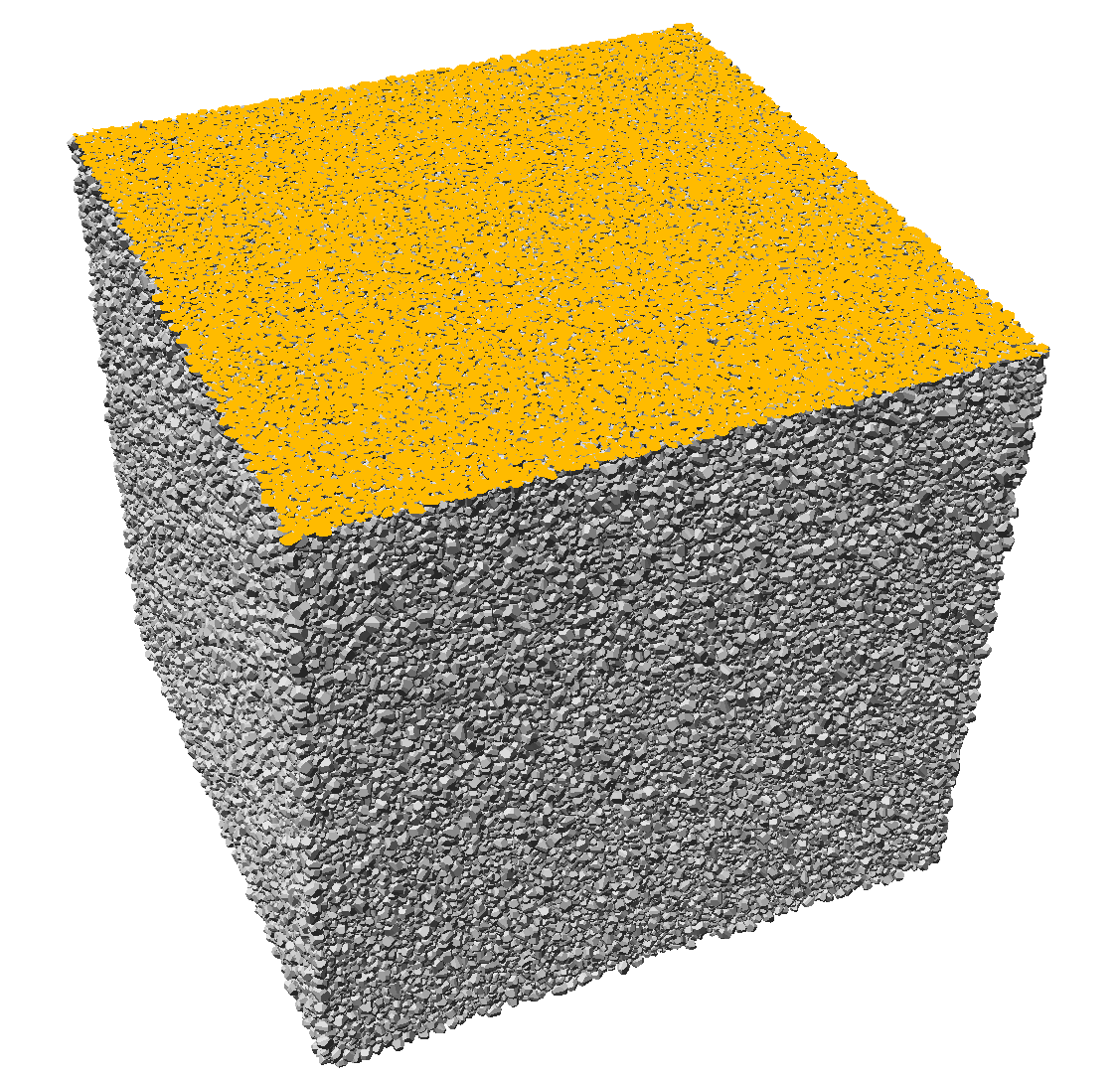
A centimeter scale porous microstructure (1.5cm sidelength)
has been discretized at nine different resolutions from submillimeter
to submicron scales covering nearly
three decades in resolution.
Our fully threedimensional microstructures may be used
for analysis, numerical computations, tests of algorithms or methods,, calibrations,
or as a starting point to generate other microstructures.
The data are available
under a Creative Commons License (CC BY-NC-SA 3.0) (Attribution-NonCommercial-ShareAlike).
size and accuracy are available to the scientific
community from this website.
A centimeter scale porous microstructure (1.5cm sidelength)
has been discretized at nine different resolutions from submillimeter
to submicron scales covering nearly
three decades in resolution.
Our fully threedimensional microstructures may be used
for analysis, numerical computations, tests of algorithms or methods,, calibrations,
or as a starting point to generate other microstructures.
The data are available
under a Creative Commons License (CC BY-NC-SA 3.0) (Attribution-NonCommercial-ShareAlike).
Our objective is to provide to the scientific public a suite of fully threedimensional digital images with a realistic strongly correlated microstructure typical for sandstone. A cubic sample of 1.5 cm sidelength (laboratory scale) has been imaged. Threedimensional (3d) images resolved at 117.18µm, 58.59µm, 29.3µm, 14.65µm, 7.3244µm, 3.662µm, 1.831µm, 0.9155µm, and 0.4576µm are available for download.
Experimental X-ray or synchrotron microtomographic (µCT) images of comparable size, resolution or data quality are, to the best of our knowledge, not available at present. Experimental µCT images of similar size and quality are not expected to become available to the scientific community for years to come. Despite the progress in fully threedimensional high resolution X-ray and synchrotron computertomography of porous media in recent years acquisition times for a 1024x1024x1024-sample of average quality at the ID19 beamline of the European Synchrotron Radiation Facility are on the order of 30 minutes. Extrapolating to the number of 32768 such blocks, that are available on this website, would require roughly 16384 hours or 2 years of uninterrupted beamtime.
|
| Three dimensional rendering of the Fontainebleau sandstone continuum model from [1]. The visualization contains over one million rendered quartz grains [7]. The image was created using the Quartz plugin for the interactive visualization software MegaMol. |
The continuum multiscale modeling technology for carbonates developed in [2] [3] [4] was applied to Fontainebleau sandstone in [1]. A laboratory sized cubic sample of sidelength 1.8cm was generated containing roughly 1.02 million polyhedral quartz grains. A visualization is seen on the right. The grains have random polyhedral shapes, they are randomly oriented and have a prescribed overlap with each other (see [1] for more modeling details). A cubic subsample of 1.5 cm sidelength was cut from the original deposit to minimize boundary effects. This sample with 1.5cm sidelength has been used for the discretizations with multiscale resolution provided on this website. Its porosity was estimated as 0.134313 and its specific internal surface area as 10 mm-1 in [0].
The model was geometrically calibrated against a well studied experimental microtomogram at 7.5µm resolution [5][6]. The geometric calibration was based on matching porosity, specific surface, integrated mean curvature, Gaussian curvature, correlation function, local porosity distributions (with 240µm and 480µm measurement cell size), and local percolation probabilities at the same measurement cell sizes. Comparison of these quantities at resolution 7.5µm was carried out in [1]. For more information on the sample see [0].
This continuum model allows the generation of synthetic micro computed tomography (s-µCT) data images at arbitrary resolution. The resulting 3D grayscale datasets, generated from discretizations at selected resolutions ranging from 458 nanometer to 117 micrometer can be used for resolution dependent high precision studies and are available to the scientific community on this page.
For details see [0], [1]. For general information on transport in porous media see [8].
References
[7] S. Grottel, G. Reina, T. Zauner, R. Hilfer, T. Ertl,
"Particle-based Rendering for Porous Media", Proceedings of
SIGRAD 2010: Content aggregation and visualization,
K. Jää-Aro and T. Larsson (eds.),
Linköping Electronic Conference Proceedings,
52, 45, 2010