II Problem and Objectives
[2.2.1.1] The problem to be discussed in this article is the
question of whether or not there exists a mesoscopic
length scale with new constitutive laws
intermediate between the microscopic pore scale ![]() and the macroscopic sample size
and the macroscopic sample size ![]() .
[2.2.1.2] Recent capillary desaturation
experiments in [11, 5] seem to hint towards
the possible existence of a mesoscopic length scale.
[2.2.1.3] Existing theories do not explain or predict
capillary desaturation curves or spatiotemporal changes
of nonpercolating residual saturations.
[2.2.1.4] Direct upscaling from pore to sample scale may be
difficult or impossible, because mesoscopic nonpercolating
clusters and their interaction become important
[12, 13, 14].
.
[2.2.1.2] Recent capillary desaturation
experiments in [11, 5] seem to hint towards
the possible existence of a mesoscopic length scale.
[2.2.1.3] Existing theories do not explain or predict
capillary desaturation curves or spatiotemporal changes
of nonpercolating residual saturations.
[2.2.1.4] Direct upscaling from pore to sample scale may be
difficult or impossible, because mesoscopic nonpercolating
clusters and their interaction become important
[12, 13, 14].
[2.2.2.1] The theoretical importance of nonpercolating trappedclusters (ganglia) on macroscopic scales (Darcy scale and larger) was emphasized already in [15]. [2.2.2.2] It was thoroughly analyzed in [16] and [17]. [2.2.2.3] The results of this analysis led to the proposal of a generalized macroscopic theory [18] predicting hysteresis [19], recovering the traditional theory [20] and highlighting the role of interfacial areas in [21]. [2.2.2.4] Experimental evidence for the possible importance of a mesoscopic scale seems to have been found in recent experimental advances allowing in situ monitoring of immiscible displacement processes using fast X-ray computed microtomography [5]. [2.2.2.5] Theoretical evidence for the importance of clusters on macroscopic scales was found in [22] for immiscible fluids in hydrostatic equilibrium, in [23] for raising and/or rotating closed columns, in [24] for Buckley-Leverett shocks, in [25] for McWhorter-Sunada flows and capillarity driven horizontal redistribution (Philip-problem), in [26] for saturation overshoot, and in [27] for multistep outflow experiments. [2.2.2.6] Recent drainage and imbibition experiments with pore scale imaging performed by [11] confirm that the dynamics is nonlocal and that capillary action at menisci is percolating over some mesoscopic distance. [2.2.2.7] These experimental observations and theoretical advances all seem to hint towards a mesoscopic cluster scale, that might be defined via the nonpercolating clusters and their interaction with each other.
[2.2.3.1] The main objectives of this paper are:
to study the process dependence of capillary desaturation curves and the dependence on the desaturation protocol,
to introduce new capillary saturation curves for oil injection based on traditional two-phase flow theory,
to propose new capillary saturation experiments with oil injection instead of water injection,
to introduce a new relation between capillary desaturation curves and the product of relative permeabilities with normalized capillary pressure functions that seems to have remained unnoticed so far,
to show that the plateau saturation in capillary desaturation curves is not given as the residual oil saturation
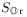 (defined in
eq. (25b) below), but can be either
(defined in
eq. (25b) below), but can be either 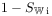 (where the irreducible water saturation
(where the irreducible water saturation 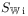 is defined in eq. (25a) below) or any saturation
below
is defined in eq. (25a) below) or any saturation
below 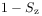 where
where 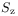 is defined as the
zero
is defined as the
zero 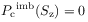 on the capillary
pressure curve for secondary imbibition, and
on the capillary
pressure curve for secondary imbibition, andto quantify the large variation of breakpoints in capillary desaturation curves within the traditional two-phase flow theory.
