4 Application Example
[387.2.1] The purpose of this section is to show that the previous theoretical framework can be used directly for predicting transport in porous media quantitatively and without free macroscopic fit parameters. [387.2.2] The theory presented above allows a quantitative micro-macro transition in porous media and opens the possibility to determine the elusive “representative elementary volume” needed for macroscopic theories in a quantitative and property specific manner.
[387.3.1] In Reference [7] a fully threedimensional experimental
sample of Fontainebleau sandstone was compared with three
geometric models, some of which had not only the same porosity
and specific internal surface area but also the same correlation
function ![]() .
[387.3.2] A large number of the geometric quantities discussed above
was calculated in [7].
[387.3.3] For a discussion on the influence contact of distrtributions see
[29].
The total fraction of percolating cells, defined in equation
(47) above, was measured in
[7] for Fontainebleau and some of its models.
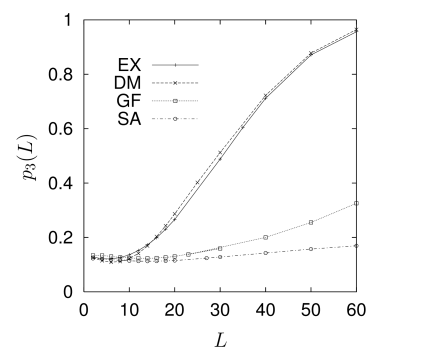
Figure 1 shows the total fraction of percolating
cells as a function of length scale (side length of
measurement cells).
.
[387.3.2] A large number of the geometric quantities discussed above
was calculated in [7].
[387.3.3] For a discussion on the influence contact of distrtributions see
[29].
The total fraction of percolating cells, defined in equation
(47) above, was measured in
[7] for Fontainebleau and some of its models.
Figure 1 shows the total fraction of percolating
cells as a function of length scale (side length of
measurement cells).
[page 388, §1]
[388.1.1] It turns out that the quantity ![]() displayed in
Figure 1 correlates very well with transport
properties such as the hydraulic permeability.
[388.1.2] Recently transport properties such as the
permesbilities and formation factors of the Fontainebleau
sandstone and its geometries were calculated
numerically exactly by solving the appropriate
microscopic equations of motion on the computer
[28].
[388.1.3] Some of the results are summarized in Table 1 below.
displayed in
Figure 1 correlates very well with transport
properties such as the hydraulic permeability.
[388.1.2] Recently transport properties such as the
permesbilities and formation factors of the Fontainebleau
sandstone and its geometries were calculated
numerically exactly by solving the appropriate
microscopic equations of motion on the computer
[28].
[388.1.3] Some of the results are summarized in Table 1 below.
| EX | DM | SA | GF | |
|
|
692 | 923 | 35 | 34 |
|
|
911 | 581 | 22 | 35 |
|
|
790 | 623 | 20 | 36 |
|
|
18.5 | 26.2 | 1.35 | 2.05 |
|
|
21.9 | 17.0 | 0.87 | 1.97 |
|
|
20.5 | 17.1 | 0.96 | 1.98 |
[388.2.1] One sees from Table 1 that while EX and DM are very similar in their permeabilities and formation factors the samples EX and GF have significantly lower values with GF being somewhat higher than SA. The same relationship is observed in Figure 1 for the percolation properties. [388.2.2] These results show that the purely [page 389, §0] geometrical local percolation probabilities correlate surprisingly well with hydraulic permeability and electrical conductivity that determine physical transport.
