4 Simulation Setup
[681.1.9.1] In this section we discuss how the experiment is represented mathematically.
[681.1.9.2] The four mass balance equations (1) are solved numerically.
[681.1.9.3] First, equations (6) and (7)
are used to eliminate ![]() and
and ![]() .
[681.1.9.4] The primary variables are
.
[681.1.9.4] The primary variables are ![]() and
and ![]() .
[681.1.9.5] The mass balances are discretized in space by cell centered finite volumes with upwind fluxes.
[681.1.9.6] They are discretized in time with a first order implicit fully coupled scheme.
[681.1.9.7] The corresponding system of nonlinear equations is solved with the Newton-Raphson method.
[681.1.9.8] The whole scheme is implemented in Matlab.
[681.1.9.9] The simulation is run with a resolution of one cell per centimeter,
i.e. with
.
[681.1.9.5] The mass balances are discretized in space by cell centered finite volumes with upwind fluxes.
[681.1.9.6] They are discretized in time with a first order implicit fully coupled scheme.
[681.1.9.7] The corresponding system of nonlinear equations is solved with the Newton-Raphson method.
[681.1.9.8] The whole scheme is implemented in Matlab.
[681.1.9.9] The simulation is run with a resolution of one cell per centimeter,
i.e. with ![]() collocation points.
[681.1.9.10] Details of the algorithm are given elsewhere [3].
collocation points.
[681.1.9.10] Details of the algorithm are given elsewhere [3].
| Parameters | Units | Values | ||
|---|---|---|---|---|
| Pa | ||||
| Pa | ||||
|
|
||||
[681.1.9.11] Dirichlet boundary conditions for the pressure ![]() of the
percolating water phase are imposed at the lower boundary (
of the
percolating water phase are imposed at the lower boundary (![]() ),
where pressure is determined by the water reservoir.
[681.1.9.12] Dirichlet boundary conditions for the atmospheric pressure
),
where pressure is determined by the water reservoir.
[681.1.9.12] Dirichlet boundary conditions for the atmospheric pressure ![]() of the percolating air
phase are chosen at the upper boundary (
of the percolating air
phase are chosen at the upper boundary (![]() ) of the column.
[681.1.9.13] All the other boundaries are impermeable so that the flux across them must vanish.
) of the column.
[681.1.9.13] All the other boundaries are impermeable so that the flux across them must vanish.
[681.1.9.14] The initial conditions are ![]() ,
,
![]() ,
, ![]() ,
, ![]() for all
for all
![]() [681.1.9.15] Initial conditions for the pressures are not required because of the implicit formulation.
[681.1.9.16] Before the protocol for the pressure is started,
the system is given one day under hydrostatic
water pressure conditions to equilibrate.
[681.1.9.15] Initial conditions for the pressures are not required because of the implicit formulation.
[681.1.9.16] Before the protocol for the pressure is started,
the system is given one day under hydrostatic
water pressure conditions to equilibrate.
[681.1.10.1] The parameters for the simulation are given in Table
1.
[681.1.10.2] They were obtained by fitting the primary drainage curve of the
capillary pressure saturation relationship obtained in
the residual decoupling approximation [9] to the
primary drainage curve of van Genuchten parametrization that
[15] obtained by a fit to data of the
first drainage process in the experiment.
[681.1.10.3] The van Genuchten parameters in [15]
are
![]() ,
, ![]() ,
,
![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
,
![]() ,
, ![]() .
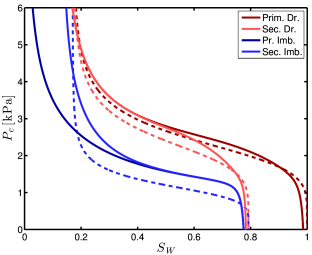
[681.1.10.4] The resulting capillary pressure curves are compared in Figure 2.
[681.1.10.5] The viscous resistance coefficients were
obtained through
.
[681.1.10.4] The resulting capillary pressure curves are compared in Figure 2.
[681.1.10.5] The viscous resistance coefficients were
obtained through ![]() ,
, ![]() , where
, where
![]() was again taken from [15].
[681.1.10.6] The viscous resistance coefficients for the
non-percolating phases are assumed to be much larger than those
for the percolating phases
was again taken from [15].
[681.1.10.6] The viscous resistance coefficients for the
non-percolating phases are assumed to be much larger than those
for the percolating phases ![]()
![]() .
[681.1.10.7] For the time-scale of the experiment
the results do not depend on the
numerical values of the resistance coefficients given in
Table 1 [3].
.
[681.1.10.7] For the time-scale of the experiment
the results do not depend on the
numerical values of the resistance coefficients given in
Table 1 [3].