Sie sind hier: ICP » R. Hilfer » Publikationen

6 Representation of the solutions as functions of time

[1287.5.1] In [20] we obtained the analytical solution of a fractional differential equation of rational order, which we use to analyze our fitting results for model A and model B. [1287.5.2] For a general solution of equations (11) and (12) with arbitrary real \alpha _{i} see [35]. [1287.5.3] The restriction to rational \alpha _{i} is not a drawback, because we can approximate \alpha _{{1}} and \alpha _{{2}} by a rational value on a grid between 0 and 1. [1287.5.4] This number of grid points is chosen to be 20, which keeps computation times reasonably limited as the computing time increases quadratically with the lowest common denominator of \alpha with 1.

[page 1288, §1]    [1288.1.1] The solution for f(t) for model B is a sum of Mittag-Leffler type functions:

f(t)=\sum _{{j=1}}^{{N}}B_{{j}}\sum _{{k=0}}^{{N-1}}c_{{j}}^{{N-k-1}}\mbox{$E\left(-k/N,c_{{j}}^{{N}};t\right)$}, (14)

where N is the smallest number for which both \alpha _{{1}}N and \alpha _{{2}}N are integers. [1288.1.2] The coefficients c_{{j}} are the zeros of the characteristic polynomial

c^{{N}}+\tau _{{1}}^{{\alpha _{{1}}}}c^{{\alpha _{{1}}N}}+\tau _{{2}}^{{\alpha _{{2}}}}c^{{\alpha _{{2}}N}}+1=0, (15)

the function E\left(\nu,a;t\right) is defined as [36]

\mbox{$E\left(\nu,a;t\right)$}=t^{{\nu}}\sum _{{k=0}}^{{\infty}}\frac{(at)^{{k}}}{\Gamma(\nu+k+1)}. (16)

[1288.1.3] The coefficients B_{{j}} are the solutions of the linear system of equations

\displaystyle\sum\limits _{{k=1}}^{{N}}c_{{k}}^{{i}}B_{{k}}=0,\qquad 0\leq i\leq N-\alpha _{{1}}N-1 (17)
\displaystyle\sum\limits _{{k=1}}^{{N}}(c_{{k}}^{{i}}+\tau _{{1}}^{{\alpha _{{1}}}}c_{{k}}^{{i-N+\alpha _{{1}}N}})B_{{k}}=0,\qquad N-\alpha _{{1}}N\leq i\leq N-\alpha _{{2}}N-1 (18)
\displaystyle\sum\limits _{{k=1}}^{{N}}(c_{{k}}^{{i}}+\tau _{{1}}^{{\alpha _{{1}}}}c_{{k}}^{{i-N+\alpha _{{1}}N}}+\tau _{{2}}^{{\alpha _{{2}}}}c_{{k}}^{{i-N+\alpha _{{2}}N}})B_{{k}}=0,\qquad N-\alpha _{{2}}N\leq i\leq N-2. (19)

[1288.1.4] This solution is only valid if all the roots c_{{j}} of the characteristic polynomial in (15) are distinct, which is checked in the computations. [1288.1.5] Because the linear system of equations (17)-(19) is underdetermined we choose one fundamental solution for \{ B_{{j}}\} and a multiplication factor for f(t) such that f(0)=1.

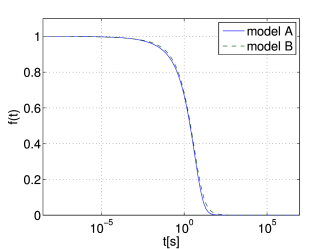
[page 1289, §1]    [1289.1.1] The analytical solutions are plotted for glycerol at 195\,{\rm K} (Fig. 7). [1289.1.2] The fitting values for \tau for model A are \tau _{{1}}=4.991\,{\rm s} and \tau _{{2}}=1.089\,{\rm s}. [1289.1.3] Both values lie in the time interval where the relaxation occurs, which confirms the interpretation of these fitting parameters as relaxation times. [1289.1.4] For model B the fitted times are \tau _{{1}}=9.729\,{\rm s} and \tau _{{2}}=0.92\,{\rm s}. [1289.1.5] So \tau _{{2}} marks the onset of the relaxation and \tau _{{1}} the end.

Figure 7: The solutions of the fractional initial value problems (6) and (7) with f(0)=1 using the fit parameters for model A and model B for glycerol at 195\,{\rm K}.

[1289.2.1] We note that the fractional derivatives appearing in the initial value problem (7) can be generalized to fractional derivatives of arbitrary type \beta introduced in [29] and defined as

\mbox{${\rm D}$}{}^{{\nu,\beta}}f(t)=\mbox{${\rm I}$}{}^{{(1-\beta)(\lceil\nu\rceil-\nu)}}\mbox{${\rm D}$}{}^{{\lceil\nu\rceil}}\mbox{${\rm I}$}{}^{{\beta(\lceil\nu\rceil-\nu)}}f(t),\qquad 0\leq\beta\leq 1. (20)

[1289.2.2] For the case \beta=1 it reduces to the Riemann-Liouville fractional derivative, while for \beta=0 to the Liouville-Caputo-type derivative [33]. [1289.2.3] Because

\mbox{${\rm D}$}{}^{{\nu,\beta}}\mbox{$E\left(\mu,a;t\right)$}=\mbox{${\rm D}$}{}^{{\nu,\gamma}}\mbox{$E\left(\mu,a;t\right)$},\qquad 0\leq\beta,\gamma\leq 1,\quad\mu>-1,\quad\nu\geq 0, (21)

the solution of our initial value problem does not change by replacing the Riemann-Liouville fractional derivatives with these generalized Riemann-Liouville fractional derivatives of type \beta.