| 1 |
M. Fisher, “The theory of crtical point singularities,” in Critical
Phenomena (M. Green, ed.), (New York), p. 1, Academic Press, 1971.
|
| 2 |
K. Binder and D. Heermann, Monte Carlo Simulation in Statistical Physics.
Berlin: Springer Verlag, 1988.
|
| 3 |
J. Cardy (ed.), Finite-Size Scaling.
Amsterdam: North-Holland, 1988.
|
| 4 |
V. Privman (ed.), Finite-Size Scaling and Numerical Simulation of
Statistical Systems.
Singapore: World Scientific, 1990.
|
| 5 |
K. Binder, “Finite size effects at phase transitions,” in Computational
Methods in Field Theory (H. Gausterer and C. Lang, eds.), (Berlin), p. 59,
Springer Verlag, 1992.
|
| 6 |
M. Barber, “Finite-size scaling,” in Phase Transitions and Critical
Phenomena VIII (C. Domb and J. Lebowitz, eds.), vol. 8, (London), p. 145,
Academic Press, 1983.
|
| 7 |
K. Binder, “Finite size scaling analysis of Ising model block distribution
functions,” Z.Phys.B, vol. 43, p. 119, 1981.
|
| 8 |
K. Binder, “Some recent progress in the phenomenological theory of finite size
scaling and application to Monte Carlo studies of critical phenomena,”
in Finite Size Scaling and Numerical Simulation of Statistical Systems
(V. Privman, ed.), (Singapore), p. 173, World Scientific, 1990.
|
| 9 |
M. Fisher, “General scaling theory for critical points,” in Collective
Properties of Physical Systems (B. Lundqvist and S.Lundqvist, eds.), (New
York), p. 16, Academic Press, 1973.
|
| 10 |
E. Brezin, “An invesitgation of finite size scaling,” J. Physique,
vol. 43, p. 15, 1982.
|
| 11 |
K. Binder, M. Nauenberg, V. Privman, and A. Young, “Finite size tests of
hyperscaling,” Phys. Rev. B, vol. 31, p. 1498, 1985.
|
| 12 |
M. Fisher, “Scaling, universality and renormalization group theory,” in Critical Phenomena (F. Hahne, ed.), (Berlin), p. 1, Springer Verlag, 1983.
|
| 13 |
V. Privman, P. Hohenberg, and A. Aharony, “Universal critical point amplitude
relations,” in Phase Transitions and Critical Phenomena (C. Domb and
J. Lebowitz, eds.), vol. 14, (London), p. 1, Academic Press, 1991.
|
| 14 |
A. Sariban and K. Binder, “Critical properties of the Flory Huggins model
of polymer mixtures,” J. Chem. Phys., vol. 86, p. 5859, 1987.
|
| 15 |
E. Brezin and J. Zinn-Justin, “Finite size effects in phase transitions,”
Nucl.Phys., vol. B257, p. 867, 1985.
|
| 16 |
A. Ferrenberg and D. Landau, “Critical behaviour of the three dimensional
Ising model: A high-resolution Monte Carlo study,” Phys.Rev. B,
vol. 44, p. 5081, 1991.
|
| 17 |
J. Cardy, “Conformal invariance,” in Phase Transitions and Critical
Phenomena (C. Domb and J. Lebowitz, eds.), vol. 11, (London), p. 55,
Academic Press, 1987.
|
| 18 |
R. Hilfer, “Thermodynamic scaling derived via analytic continuation from the
classification of ehrenfest,” Physica Scripta, vol. 44, p. 321, 1991.
|
| 19 |
R. Hilfer, “Multiscaling and the classification of continuous phase
transitions,” Phys. Rev. Lett., vol. 68, p. 190, 1992.
|
| 20 |
R. Hilfer, “Scaling theory and the classification of phase transitions,” Mod.Phys.Lett. B, vol. 6, p. 773, 1992.
|
| 21 |
R. Hilfer, “Classification theory for phase transitions,” Int.J.Mod.Phys.B, vol. 7, p. 4371, 1993.
|
| 22 |
R. Hilfer, “Classification theory for anequilibrium phase transitions,” Phys.Rev.E, vol. 48, p. 2466, 1993.
|
| 23 |
R. Hilfer, “On a new class of phase transitions,” in Random Magnetism
and High Temperature Superconductivity (W. Beyermann, ed.), (Singapore),
World Scientific Publ. Co., 1994.
in press.
|
| 24 |
R. Fernandez, J. Fröhlich, and A. Sokal, Random Walks, Critical
Phenomena, and Triviality in Quantum Field Theory.
Berlin: Springer Verlag, 1992.
|
| 25 |
D. Ruelle, Statistical Mechanics.
London: Benjamin Inc., 1969.
|
| 26 |
J. Zinn-Justin, Quantum Field Theory and Critical Phenomena.
Oxford: Oxford University Press, 1989.
|
| 27 |
B. Gnedenko and A. Kolmogorov, Limit Distributions for Sums of Independent
Random Variables.
Cambridge: Addison-Wesley, 1954.
|
| 28 |
W. Feller, An Introduction to Probability Theory and Its Applications,
vol. II.
New York: Wiley, 1971.
|
| 29 |
V. Zolotarev, “Mellin-Stieltjes transforms in probability theory,” Theor.Prob.Appl., vol. II, p. 433, 1957.
|
| 30 |
W. Schneider, “Stable distributions: Fox function representation and
generalization,” in Stochastic Processes in Classical and Quantum
Systems (S. Albeverio, G. Casati, and D. Merlini, eds.), (Berlin), p. 497,
Springer Verlag, 1986.
|
| 31 |
C. Fox, “The  and and 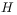 functions as symmetrical Fourier kernels,”
Trans. Am. Math. Soc., vol. 98, p. 395, 1961. functions as symmetrical Fourier kernels,”
Trans. Am. Math. Soc., vol. 98, p. 395, 1961.
|
| 32 |
A. Prudnikov, Y. Brychkov, and O. Marichev, Integrals and Series, vol. 3.
New York: Gordon and Breach, 1990.
|
| 33 | M. Cassandro and G. Jona-Lasinio, “Asymptotic behaviour of the autocovariance
function and violation of strong mixing,” in Many Degrees of Freedom in
Field Theory (L. Streit, ed.), (New York), p. 51, Plenum Press, 1976.
|
| 34 |
G. Hegerfeldt and C. Nappi, “Mixing properties in lattice systems,” Commun. Math. Phys., vol. 53, p. 1, 1977.
|
| 35 |
M. Cassandro and G. Jona-Lasinio, “Critical point behaviour and probability
theory,” Adv.Phys., vol. 27, p. 913, 1978.
|
| 36 |
Y. G. Sinai, “Mathematical foundations of the renormalization group method in
statistical physics,” in Mathematical Problems in Theoretical Physics
(G. Dell’Antonio, S. Doplicher, and G. Jona-Lasinio, eds.), (Berlin), p. 303,
Springer Verlag, 1978.
|
| 37 |
C. Domb and M. Green(ed.), Phase Transitions and Critical Phenomna.
New York: Academic Press, 1976.
|
| 38 |
F. Wegner, “Anomalous dimensions in the 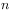 -vector model in -vector model in 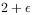 dimensions,” Z. Phys. B, vol. 78, p. 33, 1990.
dimensions,” Z. Phys. B, vol. 78, p. 33, 1990.
|
| 39 |
V. Kravtsov, I. Lerner, and V. Yudson, “Anomalous dimensions of high gradient
operators in the extended nonlinear 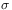 model and distribution of
mesoscopic fluctuations,” Phys. Lett. A, vol. 134, p. 245, 1989. model and distribution of
mesoscopic fluctuations,” Phys. Lett. A, vol. 134, p. 245, 1989.
|
| 40 |
G. Eyink, “Renormalization group operator product expansion in turbulence:
Shell models,” Phys. Rev. E, 1993.
|
| 41 |
B. Duplantier and A. Ludwig, “Multifractals, operator product expansions and
field theory,” Phys. Rev. Lett., vol. 66, p. 247, 1991.
|
| 42 |
K. Binder, K. Vollmayr, H. Deutsch, J. Reger, M. Scheucher, and D. Landau,
“Monte carlo methods for first order phase transitions: Some recent
progress,” Int.J.Mod.Phys.C, vol. 3, p. 1025, 1992.
|
| 43 |
A. Bruce, “Universality in the two-dimensional continuous spin model,” J. Phys. A: Math. Gen., vol. 18, p. L873, 1985.
|
| 44 |
N. B. Wilding and A. Bruce, “Density fluctuations and field mixing in the
critical fluid,” J. Phys.: Condens. Matter, vol. 4, p. 3087, 1992.
|
| 45 |
D. Nicolaides and A. Bruce, “Universal configurational structure in
two-dimensional scalar models,” J. Phys. A: Math. Gen., vol. 21,
p. 233, 1988.
|
| 46 |
C. Rickwardt, “Untersuchung der Ausschmierung einer “mean field”
Phasenumwandlung zweiter Ordnung durch endliche Systemgröße:
Das 5-dimensionale Ising Modell,” Master’s thesis, Universität
Mainz, 1993.
|
